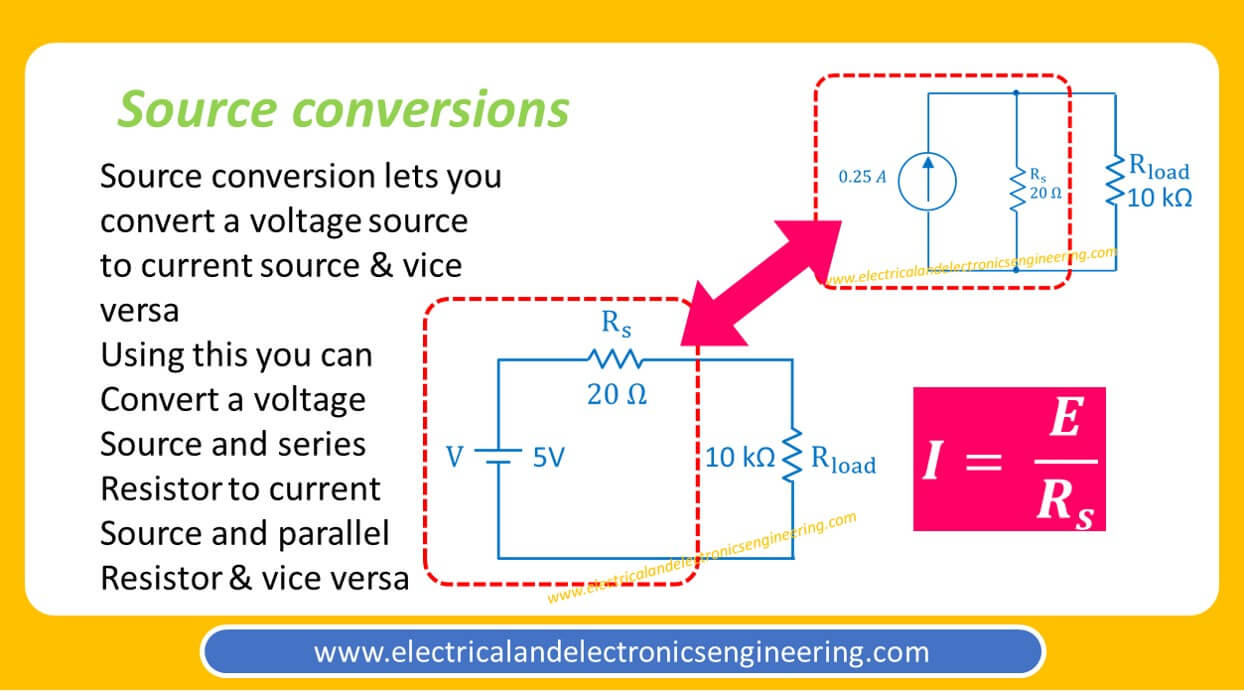
An voltage source provides constant voltage to external circuit, whereas a current source provides constant current to external circuit. A practical voltage source always contains some series resistance.
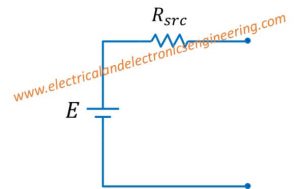
Just like voltage source, a current source also has some internal resistance, but unlike voltage source this resistance is shunt.
If we want to convert one of above mentioned source to other one we can easily do so by using the following formula:
Source conversion formulas:
- To convert current source to voltage source: E = I.Rsrc
- To convert voltage source to current source: I = E/Rsrc
An Example
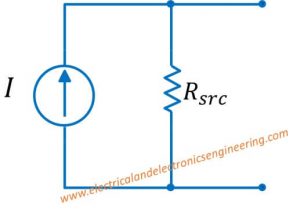
Find the equivalent current source for circuit given below. Once done find the current provided to load and prove that both sources deliver equal current to load.
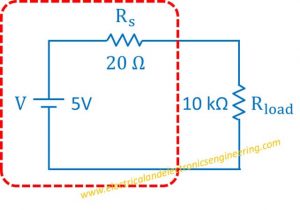
Using formula I = E/R (src) = 5 V / 20 ohms = 0.25 Amps
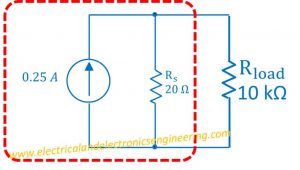
Let’s find the current provided by both sources to load.
In case of voltage source
Using Ohm’s law
I (load) = V/R = 5 V / (20 Ω + 10 kΩ) = 0.4 mA … (1)
In case of current source
Using current divider rule
I (load) = (Req/Rload) * I(s)
I (load) = (19.96/10kohm) * 0.25 A =0.4 mA … (2)
From two